Elastic Follow-up Can Result in Failures in Systems that Comply with Piping Code Rules

The analysis procedures in the Code essentially assume that the strain range in the system can be determined from an elastic analysis. That is, strains are proportional to elastically calculated stresses. The stress range is limited to less than two times the yield stress, in part to achieve this. However, in some systems, strain concentration or elastic follow-up occurs. A typical concern in refinery systems is hot walled sections in otherwise refractory lined piping systems, where thermal expansion loading has resulted in cracking in the hot walled section although it complied with the basic code acceptance criteria.
As an example, consider a cantilevered pipe with a portion adjacent to the fixed end constructed with a reduced-diameter or -thickness pipe or lower-yield-strength material that has the free end laterally displaced. The elastic analysis assumes that strains will be distributed in the system in accordance with the elastic stiffnesses. However, consider what happens when the locally weak section yields. As the material yields, a greater proportion of additional strain due to displacement occurs in the local region, because its effective stiffness has been reduced by yielding of the material. Thus, there is plastic strain concentration in the local region. In typical systems, this strain concentration is generally not considered to be significant. However, it can be highly significant under specific conditions, such as unbalanced systems. Paragraph 319.3 provides warnings regarding these conditions.
An example of a two-bar system under axial compression is provided to illustrate elastic follow-up, although the concern in piping is generally bending. However, the axial compression case illustrates the problem.
The problem is illustrated in the figure below. The elastic distribution of the total displacement, Δ, between bar A and bar B is as follows:
∆A = KB/(KA+ KB ) ∆
∆B = KA/(KA+ KB ) ∆
where
ΔA = displacement absorbed by bar
ΔB = displacement absorbed by bar B
KA = AAEA/LA elastic stiffness of bar A
KB = ABEB/LB elastic stiffness of bar B
AA = area of bar A
AB = area of bar B
EA = elastic modulus of bar A
EB = elastic modulus of bar B
LA = length of bar A
LB = length of bar B
Δ = total axial displacement imposed on two barsA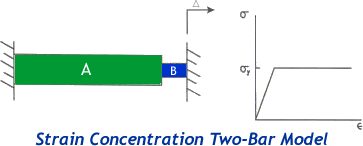
For elastic, perfectly plastic behavior of the material with a yield stress σy, the stress in bar B cannot exceed yield, so the load in bar A cannot exceed σyAB. Therefore, after bar B starts to yield, the displacement in each bar is given by
ΔAe-p = (σy AA)/KA
ΔBe-p = ∆ – ΔAe-p
where
ΔAe-p = actual displacement absorbed by A, elastic plastic case
ΔBe-p = actual displacement absorbed by B, elastic plastic case
The strain concentration is the actual strain in bar B, considering elastic plastic behavior, divided by the elastically calculated strain in bar B. Since strain is displacement divided by length, the strain concentration is given as
strain concentration = ΔBe-p /ΔB = ([∆-(σy AB/KA ) ]/LB) / ([(KA/(KA+KB ) )∆]/LB )
This can be rearranged as
strain concentration = (1+KB/KA )(1-(σy AB) / (∆KA ))
If we consider a general region under lower stress or in a stronger condition coupled with a local region under higher stress or with weaker material (e.g., lower σy), then the more flexible is the general region (stiffer is the local region), the more severe is the elastic follow-up. This can be seen by considering the above equation for strain concentration. As the stiffness of bar B increases relative to bar A, the plastic strain concentration becomes more severe.
For a system subject to plastic strain concentration, the simplest solution in design is typically to limit the thermal expansion stress range to less than the yield strength of the material. This avoids plastic strain concentration by keeping the component elastic. Although the stresses due to sustained loads such as weight and pressure usually do not need to be added to those due to thermal expansion when satisfying this limit, proper consideration of this requires a very detailed understanding of the phenomenon. Thus, it is generally preferable to conservatively add the stresses due to sustained loads to the thermal expansion stresses in this type of evaluation.
Under creep conditions, elastic follow-up can have very severe effects. This is due to the implicit assumption in the code allowable stress basis that thermal expansion stresses will relax. Near-yield-level stresses cannot be sustained very long at the very high temperatures permitted in ASME B31.3 without rapid creep rupture failures. With elastic follow-up, creep strain in the local region does not result in a corresponding reduction in thermal expansion load/stress. In severe cases of elastic follow-up, rapid creep rupture failures can occur, even though the calculated stresses fall well within Code limits. The most straightforward solution to this condition is to design the system such that the level of thermal expansion stresses in the local region subject to elastic follow-up does not exceed Sh.
A well known circumstance where elastic follow-up can occur in creep conditions is in refractory lined piping systems with local sections that are hot walled. For example, in fluid catalytic cracking units, it is common to use carbon steel pipe with an insulating refractory lining to carry high temperature fluid solids and flue gas, at temperatures to 760OC (1400OF) and higher. The metal temperature is much lower, as a result of the insulating lining. However, in some circumstances, hot wall sections such as hot wall slide valves are included in the system. The wall temperature for these components is generally the hot process temperature, so they will creep over time. In this circumstance, the thermal strain in the carbon steel portion of the system gradually transfers to the creeping hot walled section. Further, the carbon steel portion of the system acts as a spring, keeping the load on the hot walled section, preventing it from relaxing. The elastic analysis that was performed in design does not consider this behavior. Thus, piping systems that met the allowable thermal expansion stresses have cracked in service as a result of elastic follow-up. I wrote a paper in 1988, Elastic Follow-up Evaluation of a Piping System with a Hot Wall Slide Valve describing an evaluation of such a system that had failed (ASME PVP Vol-139).
As an analogous situation, consider a cantilever beam. At the built-in end, heat up the beam to creep temperatures. The elastic calculation predicts a distribution of strain over the entire beam. However, during service, the strain that was predicted to be in the low temperature portions of the beam will gradually shift to the creeping portion. Further, the low temperature portion of the beam will act as a spring, keeping a load on the high temperature portion, preventing it from relaxing.
Have a question or would like more information? You may post to this blog (below) or click the link below for more help.






Extremely very useful tips for us!!! very inciteful. I’m just leaving the comment to appreciate your efforts in disseminating the knowledge. …Thanks for the share!